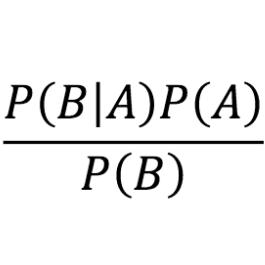Canonical Cortical Circuits and the Duality of Bayesian Inference and Optimal Control
The duality of sensory inference and motor control has been known since the 1960s and has recently been recognized as the commonality in computations required for the posterior distributions in Bayesian inference and the value functions in optimal control. Meanwhile, an intriguing question about the brain is why the entire neocortex shares a canonical six-layer architecture while its posterior and anterior halves are engaged in sensory processing and motor control, respectively. Here we consider the hypothesis that the sensory and motor cortical circuits implement the dual computations for Bayesian inference and optimal control, or perceptual and value-based decision making, respectively. We first review the classic duality of inference and control in linear quadratic systems and then review the correspondence between dynamic Bayesian inference and optimal control. Based on the architecture of the canonical cortical circuit, we explore how different cortical neurons may represent variables and implement computations.
PDF Abstract